

The general equation, in this case, becomes something like the following: This concept can be extended in the form of vector components as well. The general which can be used in this regard is as follows: If the dot product yields a zero answer, it is evident that the vectors being multiplied were actually orthogonal or perpendicular. We have already discussed that one way of finding the orthogonal vectors is by checking their dot product. Since the dot product of these 2 vectors is not a zero, these vectors are not orthogonal. Hence as the dot product is 0, so the two vectors are orthogonal.Īre the vectors a = (3, 2) and b = (7, -5} orthogonal?

Now that we know that the dot product is the major key for finding out whether the 2 vectors are orthogonal or not let’s conduct some examples for better understanding.Ĭheck whether the vectors a = i + 2j and b = 2i – j are orthogonal or not.įor checking whether the 2 vectors are orthogonal or not, we will be calculating the dot product of these vectors: Two vectors, u and y, in an inner product space, V, are orthogonal if their inner product is zero This is because the zero vector is zero and will obviously produce a null or a zero result upon being multiplied by any number or any vector. We can validate this because u.0=0 for any vector u, the zero vector is orthogonal to every vector. If we dive into the orthogonal vector properties, we get to know that the zero vector, which is basically a zero, is practically orthogonal to every vector. Hence, the dot product is used to validate whether the two vectors which are inclined next to each other are directed at an angle of 90° or not. Two vectors u,v are orthogonal if they are perpendicular, i.e., they form a right angle, or if the dot product they yield is zero. In mathematical terms, the word orthogonal means directed at an angle of 90°. What are the properties of an orthogonal vector?.In this topic, we will focus on the following areas: “2 vectors are called orthogonal if they are perpendicular to each other, and after performing the dot product analysis, the product they yield is zero.” But one of the most important concepts in this domain is the understanding of an orthogonal vector. We covered normal vectors, vector equations, vector dot products, and many others. In the realm of vector geometry, we have covered almost every concept of vectors.
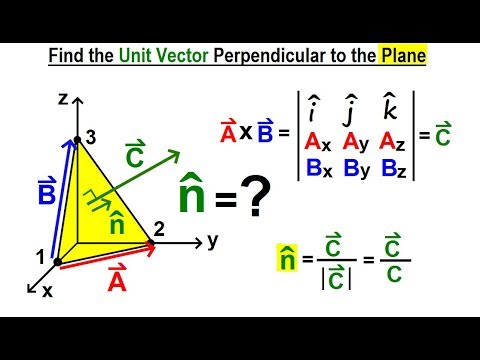
Orthogonal Vector – Explanation and Examples


 0 kommentar(er)
0 kommentar(er)
